Abduction
Peirce introduce Abduction (or Hypothesis) as an alternative to classical forms of rationality (induction, deduction). I contend that this alternative is more typical of everyday reasoning or common sense. And further, that it is a form of rationality that is particularly well suited to both the dynamics of circles and the challenges of complexity. However, my understanding of Abduction may not be representative of how many philosophers or logicians think about it.
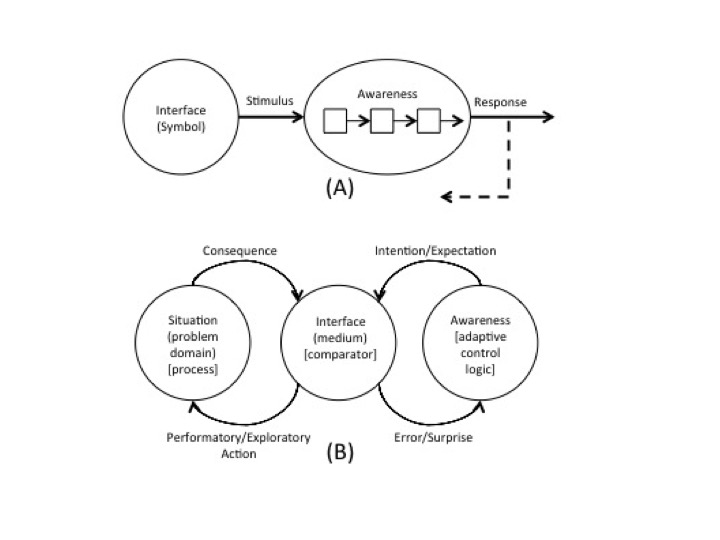
In my view, what Peirce was describing is what in more contemporary terms would be described as an adaptive control system, as illustrated in the following figure.This figure represents medical treatment/diagnosis as an adaptive control system. This system has two loops that are coupled.
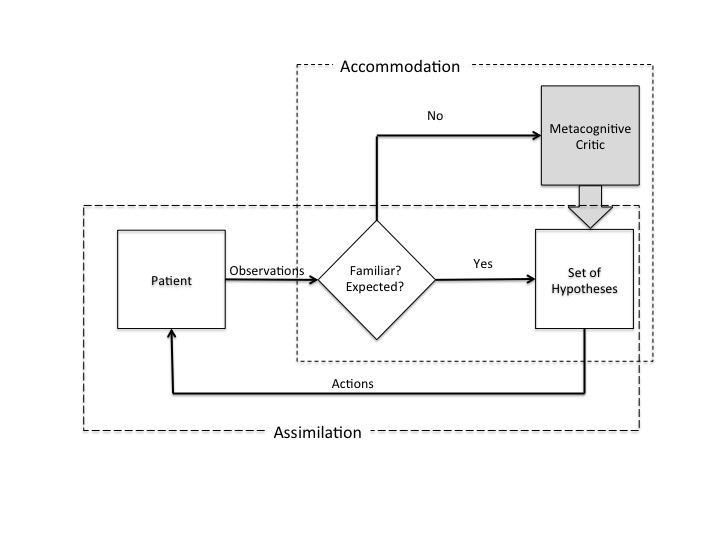
The Lower or Inner Loop - Assimilation
The lower loop is akin to what Piaget described as assimilation or what control theorists would describe as a feedback control system. This system begins by treating the patient based on existing schema (e.g., internal models of typical conditions; or standard procedures). If the consequences of those actions are as expected, then the physician will continue to follow the standard procedures until the 'problem' is resolved. However, if the consequences of following the standard procedures are 'surprising' or 'unexpected' and the standard approaches are not leading to the desired outcomes, then the second loop becomes important.
The Upper or Outer Loop - Accommodation
The upper loop is akin to what Piaget described as accommodation and this is what makes the loop 'adaptive' from the perspective of control theory. Other terms for this loop from cognitive psychology are 'metacognition' and 'situation awareness.'
The primary function of the upper loop is to monitor performance of the lower loop for deviations from expectations. Basically, the function is to evaluate whether the hypotheses guiding actions are appropriate to the situation. Are the physician's internal model or expectations consistent with the patients actual condition? In other words, is the patients condition consistent with the expectations underlying the standard procedures?
If the answer is no, then the function of the upper loop is to alter the hypotheses or hypothesis set to find one that is a better match to the patient's actual condition, In other words, the function of the upper loop is to come up with an alternative to the standard treatment plan. In Piaget's terms, the function is to alter the internal schema guiding action.
Muddling Through
The dynamic of the adductive system as illustrated here is very much like what Lindblom described as 'muddling through' or 'incrementalism.' In other words, the logic of this system is trial and error. In facing a situation, decisions and actions are typically guided by generalization from past successes in similar situations (i.e., the initial hypothesis or schema; or standard procedure). If the consequences are as expected, then the schema guiding behavior is confirmed and the experience of the physician is not of decision making or problem solving, but rather it is "just doing my job."
If the consequences of the initial trials are not as expected, then skepticism is raised with respect to the underlying schemas and alternatives will be considered. The physician experiences this as problem solving or decision making - "What is going on here? What do I try next?" This process is continued iteratively until a schema or hypothesis leads to a satisfying outcome.
This dynamic is also akin to natural selection. In this context the upper loop is the source of variation and the lower loop provides the fitness test. The variations (i.e., hypotheses) that lead to success (i.e., good fits), will be retained and will provide the basis for generalizing to future situations. When the ecology changes, then new variations (e.g., new hypotheses or schema) may gain a selective advantage.
Lindblom's term 'incrementalism' reflects the intuition that the process of adjusting the hypothesis set should be somewhat conservative. That is, the adjustments to the hypothesis set should typically be small. In other terms, the system will tend to anchor on hypotheses that have led to success in the past. From a control theoretic perspective this would be a very smart strategy for avoiding instability, especially in risky or highly uncertain environments.